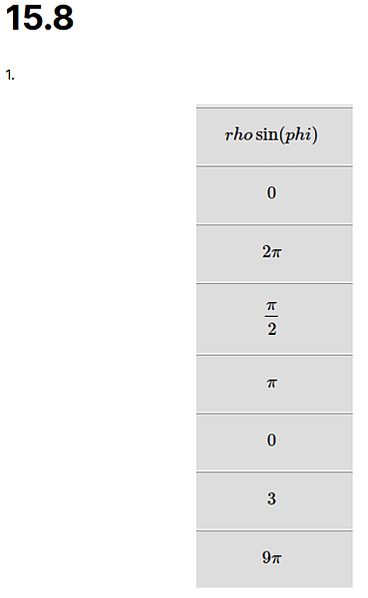
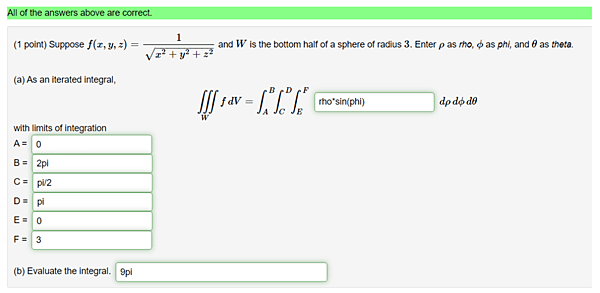

Suppose [math] and [math] is the bottom half of a sphere of radius [math]. Enter [math] as rho, [math] as phi, and [math] as theta.
(a) As an iterated integral,
with limits of integration
A =
B =
C =
D =
E =
F =
(b) Evaluate the integral.
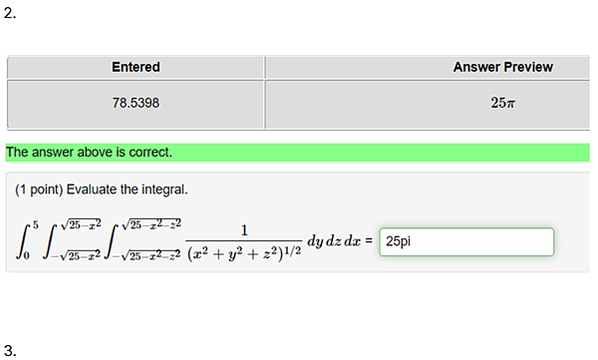
Evaluate the integral.
[math] =
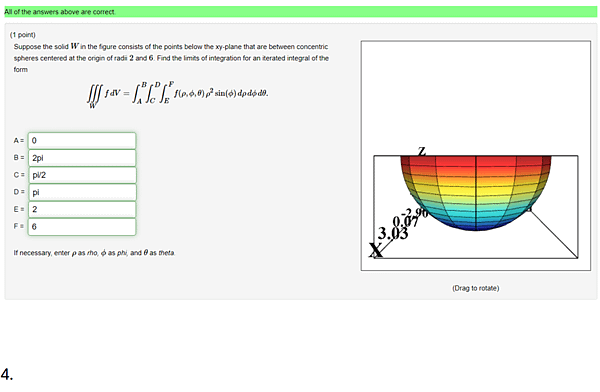
| Suppose the solid [math] in the figure consists of the points below the xy-plane that are between concentric spheres centered at the origin of radii [math] and [math]. Find the limits of integration for an iterated integral of the form
[math]
A = B = C = D = E = F = If necessary, enter [math] as rho, [math] as phi, and [math] as theta. |
(Drag to rotate) |
Note: You can earn partial credit on this problem.
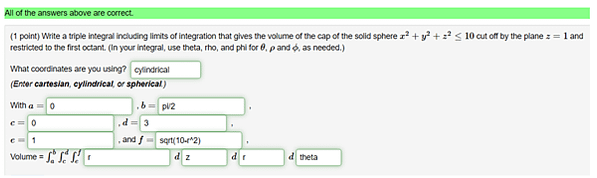
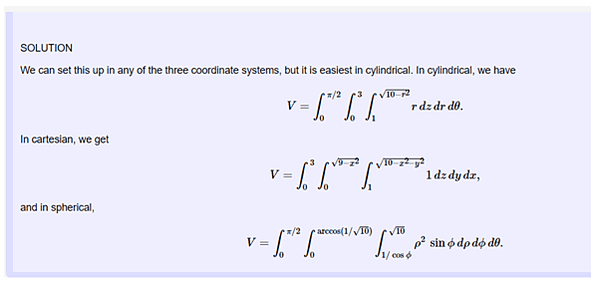
Write a triple integral including limits of integration that gives the volume of the cap of the solid sphere [math] cut off by the plane [math] and restricted to the first octant. (In your integral, use theta, rho, and phi for [math], [math] and [math], as needed.)
What coordinates are you using?
(Enter cartesian, cylindrical, or spherical.)
With [math] , [math] ,
[math] , [math] ,
[math] , and [math] ,
Volume = [math] [math] [math] [math]





 留言列表
留言列表


